This note discusses an amateur astronomy rule-of-thumb for resolving unequal binaries where the secondary is brighter than magnitude 11. Equal binaries are also discussed. Historical and current major models to predict the splitting of doubles are reviewed.

In the tetralogy of the observing act - eye, telescope, atmosphere and object - modern models tend to focus telescopic resolution performance, seeing and object intensity. By their nature, these models do not include human visual acuity. Beginning double-star observers should be aware that many modern models do not provide practical predictions for about one-half of the double stars that beginners are likely to encounter. One-half of common double star observing lists involve secondaries that are fainter than magnitude 9.0. Most modern double star split calculators do not accurately predict binary resolution where the secondary is below magnitude 9.0.

Double star resolution algorithms could be most improved by focusing future work on adding a visual acuity factor to existing telescopic performance models. If the goal is providing amateurs with practical useable information, further improvement to the accuracy of telescope performance models is of secondary importance.

Nomenclature to describe splitting doubles

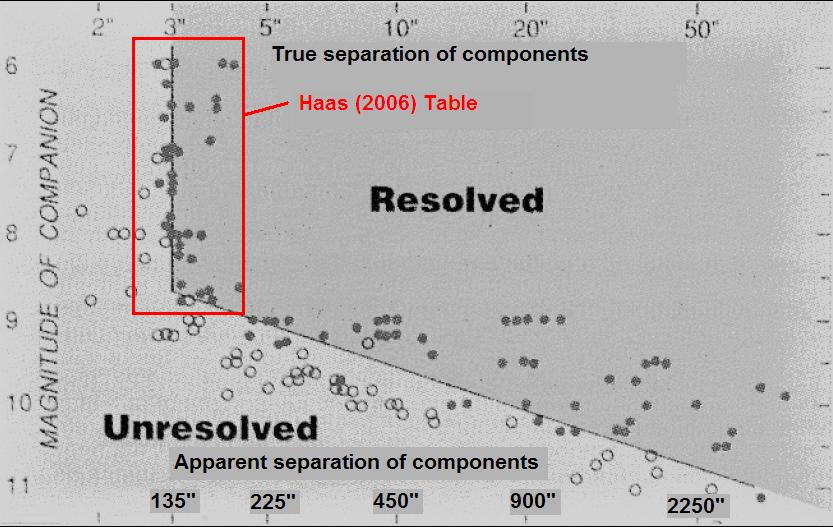
Haas (2006) suggests the following nomenclature to describe splitting doubles without numerical physical criteria, but appears to be based on the size of seeing disk. From take-offs from Haas's diagram and resolution descriptions in Rutten (2002), Table 1 suggests nomenclature for describing the degree of a binary split:

Table 1 - Nomenclature for describing double star splits after Haas (2006)

Term	Physical criteria	Graphic
Rod-shaped	~80% overlap, Dawes's criteria
Figure 8 shape	~60% overlap, Rayleigh's criteria
Touching	0% overlap, fully resolved
Hair-split	1/6 or less disk separation
Tiny gap	1/3 disk separation
Fairly close	1 disk separation
Wide	1 1/2 disk separation
Very wide	2 disk separation
Super-wide	3 disk separation
Greater than super-wide	Describe by TFOV arcsecs

In another amateur project, this author prepared a consolidated catalogue of 454 double stars from prominent double star observing lists like the Belmont Society Colorful Doubles List, the AstroLeague Double Star Observing List, Mullaney's Celestial Harvest, selected stars from the USNO 2006 Bright Double Star List, and selected stars from the 6th Orbit Catalogue. The distribution of stars in that consolidated list of doubles lists is shown as follows. 249 out of 454 (55%) secondary components are magnitude 9 or fainter.

1. Are both stars visible using a Schaefer TLM Calculator?

Equal binary rules

Equal binary rules apply where the secondary is brighter than magnitude 8.5, the difference in magnitudes is less than 2.0. In this range of conditions, your eyes use photopic-daylight vision. See discussion under "Unequal binary rule-of-thumb", below.

1. What diffraction criteria do you want to use for the split?

• Rayleigh's criteria - 140/D_mm: Applies to equal doubles measured at 550nm wavelength. Represents about a 74% dip in maximum intensity.
• Dawes's criteria - 117/D_mm: Applies to equal doubles measured at 550nm wavelength. Represents about a 3.2% dip in maximum intensity.
• Sparrow's criteria - 107/D_mm: Applies to equal doubles measured at 550nm wavelength.

For a 60mm aperture, Rayleigh's criteria is about 2.3"; Dawes is 1.9".

2. What wavelength of light to you want to measure a split in?

Traditionally this is done at 550nm, but if the star is predominately red or if you are using photographic techniques that reach down to 480nm, Rayleigh's criteria can be refigured at a different wavelength. If you drop the wavelength to 480nm, for a 60mm aperture, Rayleigh's criteria is about 2.0"

3. Test stars for equal binaries

Argyle, in his 2004 book Observing and Measuring Double Stars, filtered the Fourth Catalog of Interferometric Measurements of Binary Stars for equal doubles, yielding a list of equal binaries whose separation is known with a high degree of accuracy. The following is a subset of stars in Argyle's table ordered by right ascension. Stars selected here have known HD designations. Thus, they should be easy for the amateur to find. If this subset or Argyle's full table does not have suitable targets, the Fourth Catalogue can be accessed directly via the internet, imported into Microsoft Excel, and filtered.

Table 3 - Selected Argyle's High-Precision Equal Binary Test Stars filtered from the Fourth Catalog of Interferometric Measurements of Binary Stars

Aperture	HD_Id	Con	J2000	ADS_Id	CCDM_Id	Common	Spec	magV1	magV2	Sep	PA	Epoch
200mm	HD225220	And	J000440.10 +341554.4	32	00046 +3416	STF3056; HIP000374	K0	7.02	7.3	0.72	148	2002.0
200mm	HD002471	And	J002840.92 +371814.0	382	00287 +3718	A 1504; HIP002252	A5	8.12	8.22	0.54	28	2002.0
300mm	HD003304	Cas	J003633.60 +560833.0	504	00366 +5609	A 914; HIP002886	F5	7.97	8.05	0.46	226	2002.0
200mm	HD006886	Psc	J010939.12 +234739.8	955	01097 +2348	BU 303; HIP005444	F0	6.65	6.78	0.62	290	2002.0
400mm	HD010196	Cas	J014133.89 +624036.1	1318	01416 +6241	KR 12; HIP007895	G8V	7.81	7.88	0.37	300	2002.0
300mm	HD011126	Cas	J015119.32 +602112.2	1461	01513 +6021	A 951; HIP008629	B8V	7.98	8.26	0.45	209	2002.0
300mm	HD015328	Cet	J022759.88 +015739.2		02280 +0158	KUI 8; HIP011474	K0III	6.45	6.66	0.52	29	2002.0
300mm	HIP014255	Cet	J030348.94 -054156.8		03038 -0542	RST4220; HIP014255	F2	8.85	8.9	0.42	338	2002.0
400mm	HD019134J	Ari	J030526.71 +251518.7	2336	03055 +2515	STF 346; HIP014376	B7Vn	5.45	5.47	0.34	165	2002.0
300mm	HD026882	Cam	J041835.88 +602938.0	3105	04186 +6030	STT 75; HIP020105	B9	7.33	7.49	0.38	182	2002.0
300mm	HD029193	Tau	J043634.75 +194536.4	3329	04366 +1946	STT 86; HIP021465	A2	7.32	7.34	0.47	32	2002.0
150mm	HD031088	Ori	J045245.96 -051711.8	3497	04528 -0517	BU 316; HIP022692	F8	7.71	7.75	0.85	181	2002.0
400mm	HD034807	Aur	J052155.37 +393422.4		05219 +3934	COU2037; HIP025060	A2	7.31	7.54	0.37	141	2002.0
300mm	HD044109	Ori	J062017.14 +074308.0	4951	06203 +0743	A 2719; HIP030120	B9V	6.76	6.83	0.44	60	2002.0
090mm	HD050700	Mon	J065408.52-055108.6	5557	06541 -0551	STF 987; HIP033154	A6Vn	6.39	6.55	1.3	170	2002.0
300mm	HD055726	Gem	J071507.94 +255249.1	5918	07151 +2553	BU 1023; HIP035070	F8	8.34	8.52	0.45	298	2002.0
300mm	HD081728	Hya	J092714.57 -091325.0	1588	09272 -0913	A 1588; HIP046365	A2V	7.2	7.3	0.4	184	2002.0
200mm	HD088478	Sex	J101201.15 -061210.1	7675	10120 -0612	HO 44; HIP049961	F2	7.96	8.27	0.58	212	2002.0
300mm	HD090698	LMi	J102858.73 +345148.6	7788	10290 +3452	A 2152; HIP051320	F5	8.52	8.79	0.4	26	2002.0
200mm	HD100808	Leo	J113618.00 +274652.3	8231	11363 +2747	STF1555; HIP056601	F0V	5.8	6.01	0.71	315	2002.0
400mm	HD108005	CVn	J122424.36 +430515.0	8540	12244 +4305	STT 250; HIP060522	F0	7.88	8.02	0.35	341	2002.0
150mm	HD113415	Vir	J130346.08 -203459.2	8757	13038 -2035	BU 341; HIP063738	F7V	5.58	5.62	0.75	312	2002.0
090mm	HD114878	CVn	J131247.78 +403014.8	8820	13128 +4030	A 1606; HIP064464	K0	8.81	8.91	1.25	20	2002.0
300mm	HD115002	CVn	J131323.76 +525138.9	8825	13134 +5252	A 1607; HIP064517	G5	9.34	9.43	0.47	32	2002.0
300mm	HD134213	Lib	J150849.87 -060933.5	9515	15088 -0610	RST4534; HIP074116	F5	8.21	8.2	0.41	9	2002.0
200mm	HD136596	Ser	J152059.71 +210406.2	9600	15210 +2104	HU 146; HIP075117	G0	8.82	9.09	0.66	137	2002.0
200mm	HD137588	Dra	J152435.30 +541245.7	9628	15246 +5413	HU 149; HIP075425	K0	6.68	6.8	0.6	279	2002.0
150mm	HD192659	Cyg	J201421.58 +420614.8	13572	20144 +4206	STT 403; HIP099749	B9IV-V	7.3	7.5	0.94	173	2002.0
200mm	HD202519	Cep	J211404.85 +581750.3	14784	21141 +5818	STF2783; HIP104812	A0	7.11	7.34	0.7	15	2002.0
090mm	HD208132J	Cep	J215137.32 +654510.1	15407	21516 +6545	STF2843; HIP107893	A1m	6.37	6.67	1.4	145	2002.0
400mm	HD213530	Cep	J223032.62 +613726.0	16011	22305 +6137	HU 981; HIP111112	A0	6.98	7.23	0.29	223	2002.0
200mm	HD219617	Aqr	J231704.97 -135104.0	16644	23171 -1351	BU 182; HIP114962	F8IV	8.16	8.38	0.79	47	2002.0
300mm	HD223672	And	J235133.00 +420457.0	17050	23515 +4205	STT 510; HIP117646	A5	7.34	7.41	0.55	312	2002.0
090mm	HD223735	And	J235209.65 +433032.0	17063	23522 +4331	BU 728	F8	8.04	8.32	1.2	3	2002.0
400mm	HD224646	Cas	J235931.85 +544118.6	17151	23595 +5441	A 1498; HIP118287	F5	7.73	7.77	0.38	75	2002.0

Unequal binary rules-of-thumb - based on human visual acuity

A rule of thumb based on Peterson (1954) is summarized in Figure 1. This unequal binary rule is primarily based on the visual acuity of the human eye. It is often said that the resolution limit for the unaided eye is about 70 arcseconds or one arcminute. This applies to the ability of the eye to resolve extended line pairs - not two points. The ability of normal human vision to separate points is around 120 to 140 arcseconds. "Foveal resolution is approximately 70 [arcseconds] and for clear recognition, twice that, so the minimum magnification needed to resolve diffraction limited separations is approximately x25 per inch of aperture, the so-called Whittaker rule." Lord (1994).

If the secondary is brighter than magnitude 8.5 and the difference between the magnitudes of the primary and secondary are less than 2.0, your eyes are using photopic vision. Apply magnification necessary to raise the true separation of the double above an apparent separation of about 120" or Whittaker's rule of 25x per inch of aperture. The unequal magnitude is not strong factor. Separation generally follows the equal binary rule. If the secondary is brighter than magnitude 8.5 and the difference between the magnitudes of the primary and secondary are between 2.0 and 4.0 magnitudes, your eyes still are using photopic vision but there is a moderate effect on the eye's resolving power caused by the magnitude difference. Further detail on telescopic resolution of binaries in this magnitude range can be found Hass (2006), as summarized in Table 5 and Table 6, below. For secondaries dimmer than magnitude 8.5 and brighter than magnitude 11.5, your eyes are using scotopic, or dark-adapted, vision. As the secondaries become fainter than mag 8.5, your eye's visual acuity lessens significantly. Peterson's rule summarizes this effect where scotopic vision is used. Reading the required apparent-field-of-view (AFOV) separation from Figure 1 is the easiest method to decide what magnification is required to split a double, for apertures less than 6", Peterson (1954) provides the following equation for the required true-field-of-view (TFOV) minimum separation:

Figure 1 - Peterson's plot of resolution of binaries in 3" telescope used at 45x

Target_sep = ( 10 ^ [ 5/8 (m2 - TLM + 2.4 ) ] ) * Seeing_sep_limit {Eq. 1} where telescopic limiting magnitude is computed using the outdated form of: TLM=9.1+(5*LOG10(D_inches)) and where the seeing separation limit is the greater of 1) the smallest separation limit seen on equal binaries with the telescope-eyepiece combination, or 2) the size of the seeing disk based on current atmospheric turbulence as measured in the eyepiece in the field.

There is rough correlation between the seeing disk size and Antoniadi's scale for 8" to 20" telescopes:

Peterson's rule and Figure 1 comport with general knowledge about human visual acuity limit to separate two points - approximately 120 to 140 arcseconds.

Although reading directly from Figure 1 is the easier method, a Peterson split calculator for the secondaries with magnitudes between 8.5 to 11.5 is provided. The javascript Peterson split calculator was tested for magnitudes 9.0 through 11.0. Figure 2 plots the calculator results on Peterson's original 1954 data and indicates that this javascript implementation returns results consistent with Peterson's original algorithm. Since Peterson held magnification constant, his two-part model for bright and faint secondaries measures human visual acuity for faint stars. He also includes an atmospheric turbulence component in terms of a seeing disk.

Figure 2 - Plot of test data from javascript implementation of Peterson's algorithm over Peterson's 1954 chart

Since Peterson held magnification constant and only used a 3" telescope, his model does not adequately incorporate factor for the second player in the observing tetralogy - a telescopic performance factor. As Peterson noted, the accuracy of his scotopic performance curve is limited to 3" apertures. Peterson stated that he prepared two other curves for larger apertures and that the slope of the scotopic curve was steeper for larger apertures. This indicates that Peterson's model did not capture all relevant telescopic performance factors.

Unequal binary rule-of-thumb - based on telescope performance

Another approach to the problem of resolving unequal binaries is to focus on telescope performance instead of the response of human eye. Haas (2006) recently published a table of double split resolution limits after this technique:

Table 5 - Haas (2006) Double resolution limit table (TFOV arcsec) deltaMag Aperture (mm) 60 100 150 200 250 PrimaryLimit SecondaryLimit 0.0 2.0 1.2 0.8 0.6 0.5 4.5 4.5 0.5 2.0 1.2 0.8 0.6 0.5 4.5 5.0 1.0 2.2 1.3 0.9 0.8 0.6 4.5 5.5 1.5 2.5 1.4 1.0 0.9 0.7 4.5 6.0 2.0 3.2 1.9 1.2 1.1 0.9 4.5 6.5 2.5 3.5 2.0 1.4 1.3 1.1 4.5 7.0 3.0 3.7 2.3 1.6 1.5 1.3 4.5 7.5 3.5 4.4 2.4 1.8 1.6 1.5 4.5 8.0 4.0 4.5 2.6 2.0 1.9 1.6 4.5 8.5

Figure 3 - Overlap between Peterson's plot and Haas Table

Haas's table was based on an extraction of data from a French photometry magnitude publication, Revue des Constellations. Haas's table concerns stars brighter than magnitude 6.5 and secondaries down to 4 magnitudes fainter than the primary. The table is limited to brighter stars within those magnitude brackets - secondaries between 4.5 and 8.5 magnitudes and primaries brighter than 6.5 magnitudes. Her limits are presented in terms of the magnitude difference between primary and secondary components and the aperture of the telescope. The table excludes current seeing conditions - the size of the seeing disk - as a factor.

Assuming the "average" brightness of the primaries are 4.5 magnitudes, Haas's table correlates well with the photopic vision portion of Peterson's chart. See Figure 3, above.

Haas's tabular presentation of resolution data follows Treanor (1947). Treanor looked at resolution data for bright equal (average 4.5 mags) doubles, faint equal doubles (average 9.5 mags) and unequal doubles in those ranges using telescopes larger and smaller than 15 inches of aperture. Treanor standardized the resolution data for telescopes of differing sizes by reducing the observed split to a standard scalar. That scale index was the observed resolution divided by the Dawes limit of the telescope for equal binaries. Haas's data in Table 5 can be recast into that standardized format. In Haas's table (Table 5), the first row approximates the Dawes limit for bright equal binaries.

In the following table, the Dawes limit in the first row of each column is Table 5 used as a divisor for all cells beneath it. This standardizes the data across differing apertures:

Looking at Table 6, it is evident that there are two performance groups: telescopes less than 6 inches of aperture and greater than 6 inches of aperture. Treanor used 15 inches as the cut-off for cohort groups; Petersen noted a performance difference at 6 inches of aperture. Grouping averaging data within each 6 inch cohort gives Table 7. Table 7 is graphically presented in Figure 4, which is similar in format to Treanor's 1947 figure of telescopic performance:

Table 7 - Haas (2006) Double resolution limit - Standardized performance - grouped data deltaMag Aperture<=100(mm) >100(mm) PrimaryLimit SecondaryLimit 0 1.0 1.0 4.5 4.5 0.5 1.0 1.0 4.5 5 1 1.1 1.3 4.5 5.5 1.5 1.2 1.5 4.5 6 2 1.6 1.8 4.5 6.5 2.5 1.7 2.2 4.5 7 3 1.9 2.6 4.5 7.5 3.5 2.2 2.8 4.5 8 4 2.3 3.2 4.5 8.5

Figure 4 - Haas (2006) data recast in Treanor (1947) format - seeing limits the potential diffraction limited performance of large apertures

Treanor (1947) stands for the proposition that telescopic performance measured by aperture alone has an effect on the ability to resolve fainter secondaries that is separate from the Dawes limit.

At first glance, Figure 4 seems counter-intuitive. Why would telescopes of a larger aperture have a different performance index (have a higher resolved split / Dawes limit ratio) than smaller apertures? Why would a standardized performance index seemingly be worse in larger apertures that have a smaller Airy disks and that, in common experience, split smaller double star separations? Treanor noted that:

As the graph shows, large telescopes tend to give high values of the ratio, the implication being that they perform, on the whole, less efficiently than the smaller instruments. This last fact is not altogether surprising, since it is well known that, owing to atmospheric turbulence, large telescopes attain their theoretical limit only in exceptionally favorable weather conditions. (Treanor (1947) at 257.)

Treanor (1947) also means that any accurate prediction telescopic double star resolution performance must include a factor for atmospheric seeing.

Modern complex algorithms for splitting binaries

Modern mathematically complex models tend to focus on unifying seeing and telescopic performance into one algorithm, but by design do not include the effect of human visual acuity. As a consequence amateurs should be aware that these models are not intended to accurately model your experience of splitting doubles where the secondary is faint and dark-adapted scotopic vision is involved, i.e. - secondaries below magnitude 9.0. As noted above, that may occur over 50% of binaries that beginning double star observers are likely to encounter.

Chris Lord's Performance Index Model

Lord's performance index model is implemented in Paul Rodman's (iLanga, Inc.) Astroplanner, versions 1.58 and above.

• How it works

Chris Lord's work from the late 1990s expands on earlier work of Treanor and Peterson. Lord uses indices to represent groups of telescope and seeing characteristics. These characteristic coefficients are then used to particularize a general model of stellar object intensity. Lord's algorithm is based on the following equation:

• S = 1.033 * 10 ^ [ 1/n * ( Abs(delta mag) - 0.1 ) ] * rho {Eq.2}

where the telescopic performance index n is the sum of three indices - aperture, obstruction and seeing - from the following table with a range between 4.0 and 12.0:

Table 8 - Performance indices for Lord's algorithm

Aperture Dmm	Aperture index	Obstruction ratio	Obstruction index	Seeing - spurious disk	Seeing index
<75	4	0	4	I<0.25 rho	4
75-150	3	0.1	3	II>0.25 rho	3
151-300	2	0.2	2	III<0.50 rho	2
301-450	1	0.33	1.5	IV<1.00 rho	1
451-600	0.5	0.4	1	V>1.50 rho	0.5

where rho is Dawes criteria or rho = 116 / D_mm.

Although not explicited stated in Lord's 1994 paper, the following additional constraints are assumed:

• The primary is brighter than or equal to 9.0;
• The secondary is brighter than or equal to 13.0; and,
• The spread between the primary and secondary is at least 7.0 magnitudes.

This is the approximate magnitude domain from which Lord constructed his performance index model.

Lord has a published a nomogram - essentially a paper slide rule - by which his algorithm can be computed for a particular double, seeing condition and telescope. But the nomogram itself is difficult to use. The following are instructions for labeling Lord's nomogram and for using it. Haas (2002) also includes instructions on the use of the nomogram.

• Instructions for using Chris Lord's nomogram

1. Label the columns of the nomogram from left to right starting with A,B,C-left, C-right, D and E.
2. Determine the telescope-seeing performance index n from the Lord's index table.
3. Column E - telescope-seeing performance index n: Mark the value on Column E scale.
4. Column B - magnitude difference delta m: Mark the magnitude difference between the two stars on the Column B scale.
5. Connect the points. Read the intersecting separation performance index value from the scale Column C-right or "C - right hand side".
6. Transfer and mark the separation performance index value to the Column C-left inverse scale for (Column C - left).
7. Mark the aperture of your scope on Column D for D - aperture.
8. Draw a line through the aperture (Column D) and separation performance index value (Column C-left), extending line through Column A - predicated separation limit.
9. Read the result off of Column A, predicted separation limit (arcsecs).

• Selecting the right seeing performance index for use in Lord's nomogram

The seeing index cohorts for Lord's nomogram do not exactly follow approximate breakpoints listed in Table 4 above, taken from a Canadian Weather Service table. Amateurs typically apply a 0.5 to 1 arcsecond seeing disk in stable air to Antoniadi I, and 4 arcsecond diameter disk to AntoniadiScale IV. Lord's seeing performance index characteristic is based on a sliding seeing disk radius scale related to the diameter of the telescope's apeture. The corresponding radius and diameter is computed as follows:

rho' = n_s * (138/D) or Rayleigh's criteria - {Eq 3}.

The following calculator can give the user a feel for what seeing disk diameters viewed in their telescopes approximate Lord's performance seeing indices:

Table 9 - Antoniadi's 5 point seeing scale
Aperture (mm):

Scale	Description	Disk diameter computed from D_mm	Disk diameter for 8" (203mm) to 20" (508mm) scopes per the Canadian Weather Service	Corresponding Lord's step
I	Perfect steadiness; without a quiver.		< 0.4"	I<0.25 rho
II	Slight undulating, with moments of calm lasting for several seconds.		~ 0.4-0.9"	II>0.25 rho
III	Moderate seeing, with larger air tremors.		~ 1.0-2.0"	III<0.50 rho
IV	Poor seeing, with constant troublesome undulations.		~ 3.0-4.0"	IV<1.00 rho
V	Very bad seeing, unsuitable for anything except possibly a very rough sketch.		< 4"	V> 1.50 rho

Source: Antoniadi scale wikipedia
Canadian Weather Service. http://weatheroffice.ec.gc.ca/astro/seeing_e.html
Lord, C. Jan. 2008. Personal Communication.
Notes: Lord's seeing performance characteristic ( n_e * rho) is expressed as a radius. The third column above is twice that radius - a diameter - in order to be comparable to the Canadian Weather Service table.

• The result of Lord's performance index algorithm and poor seeing

The result of Lord's performance index algorithm gives the best possible split achievable during periods of stability, in otherwise turbulent atmospheric seeing. For the estimated minimum split using larger apetures, this can result in predicted splits that appears overly optimistic when first using the nomogram. The predicted split will be smaller than the size of the poor seeing disk. This is an intended result of alogrithm. In turbulent air and its resulting poor seeing, there are always brief moments of stability in which larger apetures can perform close to their diffraction limit, e.g. - a 400m apeture scope is estimated to split a 0.3" binary in poor 4" seeing conditions. Lord's alogrithm estimates this higher performance level during those brief periods of stability.

Lord's seeing performance index is relatively insensitive to very poor seeing (Antoniadi seeing scale IV and V).

• A javascript implementation of Lord's Performance Index Algorithm

A javascript implementation of Lord's Double Star Split Performance Index algorithm for secondaries brighter than 13.0 magnitudes has been implemented here in order to remove the computation overhead of using Lord's nomogram. The Lord Algorithm Split Calculator was tested by comparing the calculator results for a 3" refractor against the results from Lord's nomogram. Test data is based on a 3" (76.2mm) refractor, a 4.5 magnitude primary, 0% obstruction, and an Antoniadi's seeing II. The magnitude of the secondary is varied from 8.5 to 11.5. Additional calculator results for magnitudes 5.8 through 8.5 are listed for future reference.

An inherent inaccuracy in Lord's performance indices is that they are implemented in a step-wise manner. A step index is based on the average response within a class. Although computationally simplier, it overstates or understates a model's response at the end of the steps. In August 2006, Brian Tung suggested the following modifications to Lord's algorithm to make the indices continuous, instead of steps:

nD = (2.4 - (D_mm/150)) {Eq. 4} nE = (2.0 - (5*obstruction_fraction)) {Eq. 5} nS = (2.3 - (8*FWHM*(D_mm/lambda_nm))) {Eq. 6}, where FWHM is in arcseconds and D_mm and lambda_nm their unadjusted values, not reduced to common units of meters.

Figure 5 - Lord's step performance index for aperture and Tung's continuous index

These proposals were implemented in a revised calculator: Lord Performance Index Split Calculator with Tung's Continuous Performance Indices. The relative performance of each calculator in replicating the results of Lord's original nomogram are described as follows. The step-wise and continuous index calculator versions return the same results:

Table 10 - Test comparison between javascript calculator for Lord's performance index algorithm and Lord's nomogram

Primary mag	Secondary mag	delta mag	Lords's Step Calculator result	Lords's Continuous Modified Calculator	Nomogram result
4.5	5.8	1.3	2.0	2.0	n/a
4.5	6.5	2	2.4	2.4	n/a
4.5	7.5	3	3.0	3.0	n/a
4.5	8.5	4	3.8	3.8	3.8
4.5	9.5	5	4.8	4.8	4.8
4.5	10.5	6	6.1	6.1	6.3
4.5	11.5	7	7.7	7.7	8.0

Differences between the calculator and chart results are attributed to the difficulty of accurately reading and plotting points near the far ends of some scales on Lord's nomogram. Based on the above, it was felt that javascript implementation of Lord Performance Index Split Calculator with Tung's Continuous Performance Indices is an accurate implementation of Lord's algorithm.

• Astroplanner implementation of Lord's Performance Index Algorithm

Again, Lord's performance index model also is implemented in Paul Rodman's (iLanga, Inc.) Astroplanner, versions 1.58 and above. Lord's Performance Index, from Table 8, above, displays in the upper right-hand corner of the main Astroplanner display whenever a telescope and seeing condition is selected.

• Comparison of Lord's Performance Index Algorithm with Peterson (1954)

Lord's performance index model incorporates Stevens's power law. (Eq. 3, above.) Stevens's power law is a general model where human response to all stimuli - visual, pressure, temperature, motion etc. - is modeled in the form: s = k * log (I)^(a) - {Eq. 7}, where I is the intensity of the stimulation. Amateurs are familiar with Stevens's power law. Where a is approximately 0.5, Stevens's general power law is instanced as the stellar magnitude system. Arguably the Stevens's power law in Lord's performance index model means that it does incorporate a human visual acuity component. To compare Lord's performance index algorithm predictions against Peterson's empirical measurements, data in Table 10 was plotted on to Peterson's 1954 chart for a 3" telescope:

Figure 6 - Lord algorithm 3" test data plotted on Peterson's chart

Based on Figure 6, it appears that in Lord's performance index model, telescopic performance factors overwhelm any expression of human acuity response in the scotopic response range where the secondary is fainter than magnitude 9.0. Lord's model is an improved Treanor-like telescopic performance-based model (see Figure 4, above) for scotopic vision where the secondary is brighter than magnitude 9.0. In this region, Lord's algorithm is more accurate that Haas's resolving limit table (because Lord considers more of the tetralogy of key factors like seeing), but the nomogram is more difficult to use than a simple look-up table.

Chris Lord's Contrast Algorithm - another telescopic performance model

In paper originally authored in 1979, Lord explored another model for the resolution of unequal binaries - a contrast index model. Unlike his Performance Index Model that incorporates Stevens's power law, Lord's Contrast Index Model is solely a telescopic performance algorithm built around Rayleigh's criteria of telescopic resolution power. The impact of human visual acuity to resolve faint secondaries in not part of this model by design.

• Theory behind Lord's contrast model

When two Airy disks of unequal brightness overlap, the ability to resolve a split depends on two factors - (1) the relative distance between the centers of the disks and the (2) relative brightness of the disks. Combined, these two factors define the contrast between the two overlapping stars. In the case of unequal binaries, it is possible for the first diffraction ring of the brighter primary to overwhelm the full-width half-maximum (FWHM) disk. Figure 7. The lack of any difference in contrast renders the fainter secondary unresolvable. Lord sought to model the parameters of this contrast relative separation and relative intensity into two indices. The ratio of the distances between the disk centers (r1) and the size of the FWHM disk (r2) are combined into an index. The contrast between the relative brightness of the two stars (I1, I2) is combined into an index. These two indices are then used to modify a basic telescopic performance equation: Rayleigh's criteria.

Figure 7 - Schematic of two overlapping Airy disks for equal and unequal binaries

• Lord's implementation of a contrast model

Lord 1979 modifies the familiar Rayleigh criteria for telescopic performance:

sep_arsec = ( 1.22 * lambda_mm / Dmm ) radians * k_arcsec / D_mm {Eq. 8}

where k_arcsec converts from radians to arcsecs with:

k_arcsec = 360 degrees /2PI() radians * 60 arcmins per degree * 60 arcsecs per arcmin = 206265 arcsecs / radian {Eq. 9}

(Lord's paper uses focal length divided by f-ratio (f/#) to express the reciprocal of aperture in his modified Rayleigh equation. 1/D_mm is used here because it is the form more familiar to amateurs.)

Depending on wavelength of light applied and units used, gathering the coefficients in Rayleigh's criteria reduces to a few forms well known to amateurs:

sep_arcsecs = 138 / D_mm Rayleigh's criteria in millimeters {Eq. 10}

sep_arcsecs = 5.43 / D_mm Rayleigh's criteria in inches {Eq. 11}

sep_arcsecs = 116 / D_mm Dawes's criteria in millimeters {Eq. 12}

sep_arcsecs = 4.56 / D_inches Dawes's criteria in inches {Eq. 13}

These equations are in the general form:

sep_arcsecs = n / D_mm {Eq. 14}

Lord's contrast model hypothesizes that an intensity factor (F_i) and a separation factor (F_r) could be used to modify Rayleigh's criteria:

sep_arcsecs = ( F_i * F_r * n ) / D_mm Dawes's criteria {Eq. 15}

For F_i, Lord uses the relative intensity of the primary and secondary expressed using a minor modification to a well-known magnitude-brightness equation:

F_i = 100 ^ (0.1 * (Mag_secondary - Mag_primary) ) {Eq. 16}

For F_r, Lord uses:

F_r (rho') = Sqrt( 1 - kappa' ) {Eq. 17}

where kappa' = 1 / ( 1 - contrast index or gamma' ) {Eq. 18}

Lord provides other conversion questions to find gamma' from the intensity of the primary and secondary components (I1, I2) that are not repeated here.

The result is that using two parameters - the magnitude difference between the primary and secondary and aperture of the telescope - it is possible to predict the minimum separation required to resolve unequal binaries. Putting it all together reduces to Lord's Contrast Algorithm:

sep_arcsecs = (100^(0.1*deltaMag)) * 1.01 * rho' * lambda * 206265 / D_mm {Eq. 19}

Collecting coefficients in Eq. 19 allows one to generate a table in the generic from of:

sep_arcsecs = n / D_mm {Eq. 20}

- which Lord provides on pages 12 and 13 of his 1979 paper.

Lord's n coefficient table and his coefficient graph were replicated in an Excel spreadsheet, although modifications to Eq. 18 were required to Lord's method for computing kappa' in order to obtain the results shown in Lord's table on page 12:

Table 11 - Lord's Contrast Algorithm n factors with predicted minimum separations for a 3" telescope - Antoniadi I seeing assumed delta_V n Lord's_n D_mm Sep 0.0 114.6 115 76.2 1.5 1.0 168.9 169 76.2 2.2 2.0 230.4 230 76.2 3.0 3.0 303.5 302 76.2 4.0 4.0 392.8 391 76.2 5.2 5.0 503.1 499 76.2 6.6 6.0 640.6 640 76.2 8.4 7.0 812.6 804 76.2 10.7 8.0 1028.5 1016 76.2 13.5 9.0 1299.9 1282 76.2 17.1 10.0 1641.6 1646 76.2 21.5

Figure 8 - Lord's contrast model - n values by delta magnitudes - for use in sep_arcsecs = n / D_mm

With respect to incorporation of seeing in the model, Lord notes, "[i]t is also assumed the telescope is a good one and the seeing is perfect (Antoniadi I)." At pages 15, 17 and 18 of his 1979 paper, Lord suggests how the model might be supplemented for seeing. His general discussion is not expressed as an additional algrebraic term in the general contrast index model, Eq. 19.

The Excel spreadsheet reconstructing Lord's contrast index model is available for those wishing to study Lord's contrast model in more depth. (The modification required to match Lord's table is that kappa' was found from 1/gamma' and not 1/(1-gamma'). All of the coefficients computed in Table 10 match Lord's computed coefficients to less than +-1%.)

Lord's n coefficients have the appeal of simplicity. Look-up the magnitude difference of your double's components, find the n coefficient and divide by your aperture.

• Comparison of Lord's Contrast Index Model with Peterson (1954)

Like his Telescopic Performance Index algorithm, Lord's Contrast Index Model Lord focuses on telescopic performance. By design it does not include a factor for the reduction of visual acuity due to scotopic vision where the secondary is less than magnitude 9.0.

Figure 9 - Lord's contrast model plotted against Peterson (1954)

Luis Arugelles's LIDAC model

Luis Arugelles developed fuzzy logic software, "Luis Argüelles Difficulty Index Calculator (LIDAC), which returns a difficulty index for splitting doubles. This algorithm is based on neural net logic and not physics or human visual physiology, so it is not reviewed here. Like Chris Lord's Performance Index, LIDAC is also implemented in Paul Rodman's (iLanga, Inc.) Astroplanner. Arugelles' LIDAC is worth downloading and trying - either in his original application or in Rodman's Astroplanner. Arugelles also has an extensive double star amateur astronomy project called "The Spirit of 33". The "The Spirit of 33" project is a catalogue of 33 doubles in each of the 88 known constellations.

Is the future of double split algorithms in improving prediction of telescopic resolution or in adding a visual acuity factor?

An double split algorithm that would have the most practical value to an amateur is one that incorporates all of the key factors in the tetralogy of the observing event, e.g. in the form:

sep_arcsecs = F_v * F_tp * F_seeing * F_obj {Eq. 21}

where -

sep_arcsecs = F_v = human visual acuity factor {Eq. 22}

sep_arcsecs = F_tp = telescopic performance factor {Eq. 23}

sep_arcsecs = F_seeing = atmospheric turbulence or seeing factor {Eq. 24}

sep_arcsecs = F_obj = object intensity factor {Eq. 25}

Formulation of a visual acuity factor is beyond this amateur author's ability. Schaefer (1990) did considerable work on this factor in formulating his telescopic limiting magnitude algorithm. However for demonstration purposes, a simple visual acuity factor based on a reciprocal Stevens's power law can be used to approximate such a factor and shows how it might improve the utility of modern double splitting algorithms to amateurs. In this demonstration example, Lord's performance index algorithm is used to capture telescopic performance, seeing and object intensity:

sep_arcsecs = F_v * [ Lord's Performance Index Calculator ] {Eq. 26}

where, the four factor - visual acuity is captured using a reciprocal Stevens's power law:

F_v = (1 + (0.0001064 * (1 / 10^( magSecondary / - 2.5 ))) {Eq. 27}

The effect of the visual acuity or scotopic vision factor on Lord's Performance Index model is shown by table and chart as follows:

Table 11 - Demonstration of scotopic vision factor applied to 3" telescope to predict the minimum separation in arcsecs needed to resolve a double magPri magSec deltaMag Lord_PIM F_v Minimum_sep 4.5 5.8 1.3 2 1.0 2.0 4.5 6.5 2 2.4 1.0 2.5 4.5 7.5 3 3 1.1 3.3 4.5 8.5 4 3.8 1.3 4.8 4.5 9.5 5 4.8 1.6 7.8 4.5 10.5 6 6.1 2.6 15.7 4.5 11.5 7 7.7 4.9 38.1

Figure 10 - Lord's performance index model modified with a scotopic visual acuity factor plotted against Peterson (1954)

Testing your own eye's resolution

It is also useful to test the resolving ability of your eyes to rule them out as a factor in splitting doubles. Although stars are point objects, you can test the resolving power of your eyes against line charts and the normal human eye linear resolving power of approximately 70 arcseconds. The References section includes resources to obtain, download and print either USAF 1951 line resolution charts or the Koren 2003 Lens Test Chart. Print one of the charts using the highest resolution available on your printer and tape the chart about 8-10 feet away to a wall. Measure the distance from the chart to your chair. Your objective is to determine the angular size of the smallest resolvable black and white line-pair. Use the half angle formula to determine the angular size of one white and black line pair. This will only give a rough measurement of the resolving ability of your eyes, since the charts are printed on a laser printer, instead of plastic film.

Disclaimers

This note is amateur astronomer product. Corrections to any errors are welcomed and appreciated.

References:

Arguelles, Luis. 2006. The Spirit of 33 Project. (Website). The Spirit of 33

Arguelles, Luis. 1999. Fuzzy Splitting. (LADIC software). http://www.carbonar.es/s33/Fuzzy-splitting/fuzzy-splitting.html

Argyle, R. (ed). 2004. Tables 2.1-2.7, Test Stars for Binocular through 600mm Telescopes. In Observing and Measuring Visual Double Stars. Springer. ISBN 1-85233-558-0 http://www.springer.com/

Haas, S. Jan. 2002. Enjoying Unequal Double Stars. Sky & Telescope (Lord's nomogram)

Haas, S. 2006. Double Stars for Small Telescopes. Sky Publishing. pp. 5-6

Hartkopf, William I., Brian D. Mason, & Gary L. Wycoff (USNO). Sept. 2005. Fourth Catalog of Interferometric Measurements of Binary Stars. (Webdatabase) http://ad.usno.navy.mil/wds/int4.html

Koren, Norman. 2006. Understanding Image Sharpness. (Koren 2003 lens test chart). http://www.normankoren.com/Tutorials/MTF5.html

Lord, Chris. 1979 (Rev. ____). Contrast & Definition. Brayebrook Observatory. http://www.brayebrookobservatory.org/BrayObsWebSite/BOOKS/contrast%26definition.pdf

Lord, Chris. 1994. Nomogram for Telescopic Resolution of Unequal Binaries. Brayebrook Observatory. http://www.brayebrookobservatory.org/BrayObsWebSite/HOMEPAGE/BRAYOBS%20PUBLICATIONS.html

Lord, Chris. 1994. A Report on the Analysis of the Telescopic Resolution of Unequal Binaries. Brayebrook Observatory. http://www.brayebrookobservatory.org/BrayObsWebSite/BOOKS/TELESCOPIC%20RESOLUTION.pdf

Lord, Chris. Jan. 2008. Personal Communication.

Monaghan, R. Lens. Undated. Resolution Testing. (USAF 1951 Test Charts) http://medfmt.8k.com/mf/resolution.html

Peterson, H.H. Sept. 1954. S&T. p. 396

Rodman, Paul, iLanga, Inc. 2007. Astroplanner (software)

Schaefer, B.E. Feb. 1990. Telescopic Limiting Magnitude. PASP 102:212-229 http://adsbit.harvard.edu/cgi-bin/nph-iarticle_query?bibcode=1990PASP..102..212S

Tung, Brian. 8/14/2006. Personal Communication

Treanor, P.J. 1946. On the Telescopic Resolution of Unequal Binaries. The Observatory. 66:265. http://adsabs.harvard.edu/cgi-bin/nph-bib_query?bibcode=1946Obs....66..255T

_________. ____. USAF 1951 Test Chart. http://members.cox.net/lenstestr1/AIRFORCECHART.jpg

Content-Child Safe Ratings

•